Question

The two lines, P and Q, are graphed below: Line P is drawn by joining ordered pairs negative 8,15 and 6, negative 12. Line Q is drawn by joining ordered pairs 4,16 and negative 9,10 Determine the solution and the reasoning that justifies the solution to the systems of equations

Answer 1

(−2, 4), because it is the point of intersection of the two graphs

Explanation:

BECAUSE

Answer 2

`\text{Solution: }\left(-(2654)/(435),(1644)/(145)\right)`

Explanation:

Line P is drawn by joining ordered pairs (-8,15) and (6,-12)

Using two point formula of line. Find equation of line P

`(y-15)/(x-(-8))=(-12-15)/(6-(-8))`

`(y-15)/(x+8)=(-27)/(6+8))`

`(y-15)/(x+8)=(-27)/(14)`

`y=(-27)/(14)(x+8)+15`

`y=(-27)/(14)x+(-27)/(14)* 8 + 15`

`y=(-27)/(14)x-(3)/(7)`

`\text{Equation of line P:}y=(-27)/(14)x-(3)/(7)`

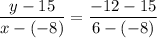
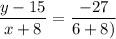
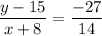
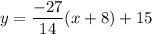
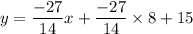
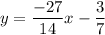
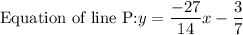
Line Q is drawn by joining ordered pairs (4,16) and (-9,10)

Using two point formula of line. Find equation of line Q

`(y-16)/(x-4)=(10-16)/(-9-4)`

`(y-16)/(x-4)=(-6)/(-13)`

`(y-16)/(x-4)=(6)/(13)`

`y=(6)/(13)(x-4)+16`

`y=(6)/(13)x-(6)/(13)* 4 + 16`

`y=(6)/(13)x+(184)/(13)`

`\text{Equation of line Q:}y=(6)/(13)x+(184)/(13)`

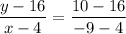
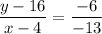
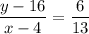
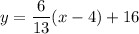
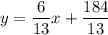
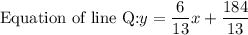
Using graphing find solution of system of equation.

We draw the line on graph and see the intersection point.

Please see the attachment of the line.