Question

Find the 12th term in the following geometric sequence. 0.75, 1.5, 3, 6, . . .

Answer 1

the pattern doubles, .75 x 2= 1.5. 1.5 x 2= 3, and so on. so the answer would be 1,536

Answer 2

1536

Explanation:

Given : A geometric sequence 0.75, 1.5, 3, 6, . . .

To Find:Find the 12th term

Solution:

0.75, 1.5, 3, 6, . . .

First term =a = 0.75

Common difference =
`r = (a_3)/(a_2) =(a_2)/(a_1)=(1.5)/(0.75)=(3)/(1.5)=2`

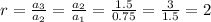
nth term of G.P. =
`a_n=a r^(n-1)`

Where a = First term = 0.75

r = common difference = 2

Substitute n = 12

`a_(12)= 0.75 (2)^(12-1)`

`a_(12)= 0.75 (2)^(11)`

`a_(12)= 1536`

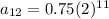
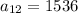
Hence the 12th term in the geometric sequence. 0.75, 1.5, 3, 6, . . . is 1536.