Part 1We are given

. This can be rewritten as
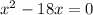
.
Therefore, a=1, b=-18, c=0.
Using the quadratic formula
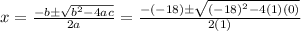
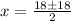
The values of x are
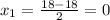
 Part 2
Part 2Since the values of y change drastically for every equal interval of x, the function cannot be linear. Therefore, the kind of function that best suits the given pairs is a
quadratic function. Part 3.The first equation is
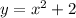
.
The second equation is
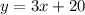
.
We have
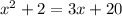
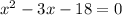
Factoring, we have
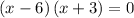
Equating both factors to zero.
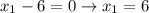
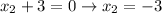
When the value of x is 6, the value of y is
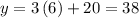
When the value of x is -3, the value of y is
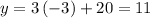
Therefore, the solutions are (6,38) or (-3,11)