Answer:
a,b and c.
Explanation:
We have to find the the functions that are their own inverses.
a.t(p)=p
Then the inverse function of given function is
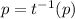
Therefore, the given function is inverse function of itself.
Hence, option a is true.
b.y(j)=
![-(1)/(j)</p><p>Let y(j)=y then we get </p><p>[tex]y=-(1)/(j)](https://img.qammunity.org/2019/formulas/mathematics/college/xgzommcxqplx5yf6mow5nb5lohhjhynp3j.png)


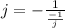
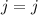
Hence, the function is inverse of itself.Therefore, option b is true.
c.
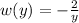
Suppose that w(y)=w
Then
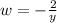
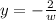
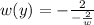
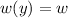
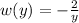
Hence, the function is inverse function of itself.Therefore, option c is true.
d.
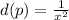
Let d(p)=d
If we replace
![(1)/(x^2)by p then we get </p><p>[tex]d=(1)/(x^2)](https://img.qammunity.org/2019/formulas/mathematics/college/y8jhhp0zf0rqcho97724h69nmllcc193bh.png)

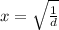

Hence, the function is not self inverse function.Therefore, option d is false.