Question

What are the vertical and horizontal asymptotes for the function f(x)=x^2+x-6/x^3-1

Answer 1

vertical asymptote at x = 1

horizontal asymptote at y = 0

Explanation:

`f(x)=(x^2+x-6)/(x^3-1)`

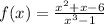
WE factor both top and bottom

x^2 + x-6 can be factored as (x+3)(x-2)

Now we factor x^3 -1

x^3-y^3= (x-y)(x^2+xy+y^2)

x^3 - 1^3=(x-1)(x^2+x+1)

replace the factors

`f(x)=((x+3)(x-2))/((x-1)(x^2+x+1))`

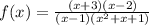
To find vertical asymptote , we set the denominator =0 and solve for x

x^3 -1 =0

x^3 = 1

Now take cube root

x = 1

So vertical asymptote at x=1

To find horizontal asymptote we look at the degree of numerator and denominator

Degree of numerator =2 and degree of denominator = 3

Degree of numerator is smaller than the degree of denominator so horizontal asymptote at y=0