So you just need to set up the limit definition.
You would need to use the formula

Where
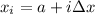
and
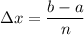
.
Basically we would have approximate area with

rectangles with width
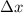
and height
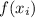
, and we have the value of n approaches to infinity.
Now, from the definite integral, we have
.
So then that would be
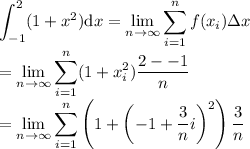
So this is how you set up the limit definition of definite integrals.
Hope this helps.