To find this, we need to find the Surface area of the lateral surface of the cone (without the circle base).
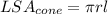
Now we just need to plug what we know into the equation
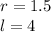

Wither you can keep your answer in pi or take it out of pi, depending on what the requirements are.
To take it out of pi you need to multiply 6 by pi (remembering to use 3.14 as pi in most cases since that is what is generally accepted on tests and exams).
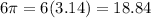
Then rounding to the nearest square inch which would mean that the amount of paper needed is =19in²