Answer:
If n decreases to one or zero, the expression will converge to 16 or 15, but if n decreases to minus infinity, the expression will diverge to minus infinity. The diference between the value of the expression, and the value of n, is always 15.
Explanation:
To explain this result, we have to treat the expression as a succession, which we can write as
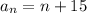
then, to analyze it, we have to know which type of number is n (but the problem doesn't tell us).
In mathematics, in general, n is used to name a natural number (this is, n=1, 2, 3, 4, ...), but sometimes n is a natural number plus zero (this means n=0, 1, 2, 3, ...). Nevertheless, in this problem it is not said which type of number n is, therefore it could be an integer too (this means n=..., -2, -1, 0, 1, 2, ...). And we will stop there and not say that n could be real, fractional, irreal, etc. (which could be, as it is not defined in the problem).
Therefore, as n is decreasing, we will take the limit of n decreasing to its three possible versions:
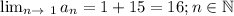
if n is natural,
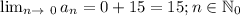
if n is natural plus zero, and
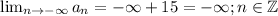
if n is an integer.
So, these are the correct answers to the general expression given in the problem.