Question

Cos2a= 2cos^2a-1 for all values of a

true or false?

Answer 1

true
Cos(A+B)=CosACosB-SinASinB
therefore Cos(A+A)= CosACosA - SinASinA
= Cos^2A - Sin^2A

Answer 2

For the solution/answer of this question, we will use the double angle formula which can be derived as follows:

`cos(2a)=cos(a+a)=cos(a)* cos(a)-sin(a)* sin(a)=cos^2(a)-sin^2(a)`

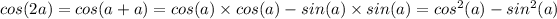
Now, the above expression can be simplified to:

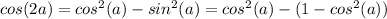`cos(2a)=cos^2(a)-sin^2(a)=cos^2(a)-(1-cos^2(a))` (Because
`sin^2a=1-cos^2a`)

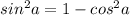Therefore, we can further simplify it to:

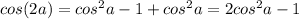`cos(2a)=cos^2a-1+cos^2a=2cos^2a-1` which is the proof which is required.

Thus, we have proved that:

`cos(2a)=2cos^2a-1`

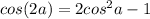
Thus, this equality is true for all values of a.