Short answer <<<< C
The length of an arc is given by the formula

Since the arc length is given (4
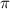
) and the radius is given (6), the central angle

can be found
Arc Length = theta * r
4 pi = theta * 6 Divide by 6
4 pi / 6 = theta
theta = (2/3) pi <<<<<< answer.
Answer C <<<<<<
answer.