Question

(08.01)

Given the arithmetic sequence an = 4 − 3(n − 1), what is the domain for n?

All integers where n ≥ 1
All integers where n > 1
All integers where n ≤ 4
All integers where n ≥ 4

answer by 1:25 EST for an extra 30 points!!

Answer 1

All integers where n ≥ 1

Explanation:

Given :
`a_n = 4 - 3(n - 1)`

To find: Domain for n

Solution:

Arithmetic sequence is a sequence of numbers such that the difference between the consecutive terms is constant. Difference here means the second minus the first.

In arithmetic sequence,
`a_n=a+(n-1)d`

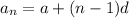
where a is first term.

d is common difference.

n is number of terms which belongs to natural numbers

And according to the definition of Arithmetic sequence n starts with 1

And we also know that natural number starts with 1

Now, in given sequence for nth term :
`a_n = 4 - 3(n - 1)`

The domain for n is All integers where n ≥ 1

Answer 2

`a_n=4-3(n-1)\\\\n\in\mathbb{N^+}\\\\Answer:\ \text{All integers where}\ n\geq1`