Question

Change the fraction into an equivalent fraction with the denominator w2 + w – 20.

Answer 1

Answer:

`((w-3)(w-4))/((w+5)(w-4))`

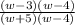
If we want to expand the brackets, the fraction would be:

`(w^2 - 7w + 12)/(w^2 + w - 20)`

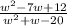Step-by-step explanation:
First, we can note that:
w² + w - 20 can be factorized as follows:
w² + w - 20 = (w+5)(w-4)

The given expression already has a (w+5) in the denominator, therefore, all we need to do is multiply the denominator by (w-4)

Since we want the new fraction to be equivalent to the original one, therefore, as we multiply the denominator by (w-4), we will also multiply the numerator by (w-4) to ensure that the value of the fraction is unchanged.

Based on the above, the new fraction would be:

`((w-3)(w-4))/((w+5)(w-4))`

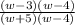
If we want to expand the brackets, the fraction would be:

`(w^2 - 7w + 12)/(w^2 + w - 20)`

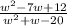
Hope this helps :)