Answer: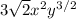 This can be rewritten as:
This can be rewritten as: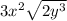 Step-by-step explanation:Before we begin, remember the following:
Step-by-step explanation:Before we begin, remember the following:√ab = √a * √b
√xᵃ = x^(a/2)
Now, for the given, we have: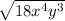
18 can be rewritten as 9 * 2
Therefore, the given expression would now become:
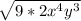
Distributing the root as mentioned in the above rules, we would get:
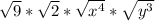
We know that:

(we would disregard the negative value of the root)
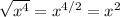
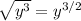
Substitute with the above in the expression, we would get the final answer:
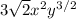
This can be rewritten as:
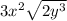
Hope this helps :)