The wavelength (A) of a photon of green light is 521 nm
To calculate the wavelength
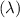 of a photon of green light with a given frequency (v), we use the formula that relates wavelength, frequency, and the speed of light (c):
of a photon of green light with a given frequency (v), we use the formula that relates wavelength, frequency, and the speed of light (c):
![\[ c = \lambda * v \]](https://img.qammunity.org/qa-images/2023/formulas/chemistry/high-school/komccg6x6pnwyo8savof.png)
Where:
- c is the speed of light in a vacuum, approximately
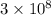 meters per second (m/s).
meters per second (m/s).
-
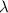 is the wavelength in meters (m).
is the wavelength in meters (m).
- v is the frequency in hertz
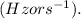
Given:
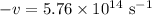
The speed of light c is a constant at
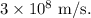
Now, to find the wavelength
 we rearrange the formula to solve for \
we rearrange the formula to solve for \
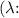
![\[ \lambda = (c)/(v) \]](https://img.qammunity.org/qa-images/2023/formulas/chemistry/high-school/r3naeu4stnt5122ou21i.png)
Substitute the given values into the equation:
![\[ \lambda = \frac{3 * 10^8 \text{ m/s}}{5.76 * 10^(14) \text{ s}^(-1)} \]](https://img.qammunity.org/qa-images/2023/formulas/chemistry/high-school/qaqtd9lydga2p17c4iey.png)
Next, we will calculate the value of
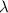 and convert it to nanometers (nm) because
and convert it to nanometers (nm) because
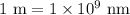 . Let's perform the calculation.
. Let's perform the calculation.
The wavelength
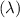 of the photon of green light with a frequency
of the photon of green light with a frequency
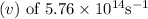 is approximately 521 nanometers nm when rounded to the nearest whole number.
is approximately 521 nanometers nm when rounded to the nearest whole number.