Answer:
OptionB is right
Explanation:
Given that an ellipse centered at the origin with vertices (8,0) and (-8,0) and a minor axis length of 8
Since centre is at the origin, its equation would be of the form
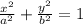
Since vertices are (8,0) and (-8,0) we find that a=8
Minor axis length = 2b =8 (given)
So b =4
The equation of the ellipse would be
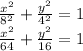
Option B is the right answer