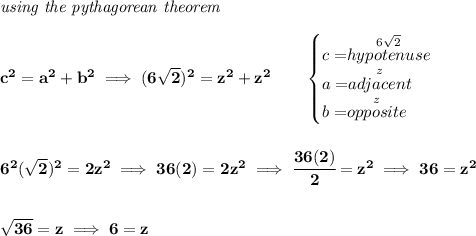
there are a couple of ways to tackle this one, using the 45-45-90 rule or just using the pythagorean theore, let's use the pythagorean theorem.
the angle at A is 45°, and its opposite side is BC, the angle at C is 45° as well, and its opposite side is AB, well, the angles are the same, thus BC = AB.
hmmm le'ts call hmmm ohh hmmm say z, thus BC = AB = z.