Answer: The correct option is
(A)

Step-by-step explanation: We are given to select the correct summation form that represents the sum of the following series :
6 + 8 + 10 + 12 + 14 + 16 + 18 + 20 + 22 + 24 + 26.
We see that
the first term of the series, a = 6.
And, there is a common difference in any two consecutive terms given by
d = 8 - 6 = 10 - 8 = . . . = 2.
This implies that the given series is arithmetic with first term a = 6 and common difference d = 2.
We know that
the sum of first n terms of an arithmetic series with first term a and common difference d is given by
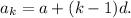
Since there are 11 terms in the given series, so the required sum is given by
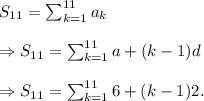
Thus, (A) is the correct option.