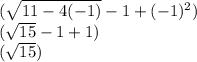The correct answers are:
(1) 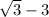
↔ (g+f)(2)
(2) 0
↔ (
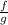
)(-1)
(3) 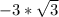
↔ (g x f)(2)
(4) 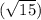
↔ (g-f)(-1)
Step-by-step explanation:
Box-1:Step 1: Find (g + f)

--- (1)
Step 2: Now Plug in x = 2 in (1) gives you (g+f)(2):
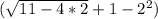
=>
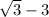 Box-2:
Box-2:Step 1: Find (
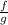
)

--- (2)
Step 2: Now Plug in x = -1 in (2) gives you (
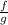
)(-1):
 Box-3:
Box-3:Step 1: Find (f x g)
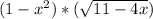
--- (3)
Step 2: Now Plug in x = 2 in (3) gives you (g x f)(2):
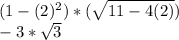 Box-4:
Box-4:Step-1: Find (g-f):
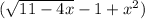
--- (4)
Step 2: Now Plug in x = -1 in (4) gives you (g-f)(-1):