Question

A road has an incline of 10°. To the nearest foot, find the increase in altitude of a car after driving 4,000 feet along the road. A) 695 ft B) 705 ft C) 3,939 ft D) 4,062 ft

Answer 1

A.

You can get this by creating a right triangle with a opposite side of x and the hypotenuse of 4000. Then find the altitude using Sin(10) = x/4000. Solve for x.

Answer 2

(A) 695ft

Explanation:

Given: A road has an incline of 10°.

To find: The increase in altitude of a car after driving 4,000 feet along the road.

Solution: It is given that A road has an incline of 10°, thus using the trigonometry, we have

`(AB)/(AC)=sin10^(\circ)`

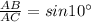
Substituting the given values, we get

`(x)/(4000)=sin10^(\circ)`

`(x)/(4000)=0.174`

`x=4000(0.174)`

`x=694.5ft`

`x`≈
`695ft`

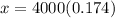
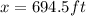
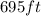
Thus, the increase in altitude of a car after driving 4,000 feet along the road is 695 feet.

Hence, option A is correct.