The formula to find the amount is

here A is amount
P is the principal
'r' is the rate of interest
n is the number of years.
Case 1.
Stevan invests
P =$ 20,000
r = 3% = 0.03
n = 10 years
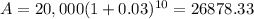
Hence the interest earned
= A - P = 26878.33 - 20000 = $6878.33
Case 2.
Evan invests
P = $10,000
r = 7% = 0.07
n = 7 years
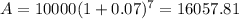
Hence the interest earned
= A - P = 16057.81 - 10000 = 6057.81
Difference in the interest = 6878.33 - 6057.81 = $820.52
Rounded to the nearest dollar difference in interest = $821