For any trigonometric point P(x,y)
x always represents cos
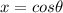
y always represents sin.
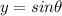
Now if we drop a perpendicular from P(x,y) to a point Q which is a refelction of P across x axis, we get Q(x, -y) for the same angle.
The angle shall be
So now
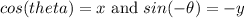 ...(1)
...(1)
But
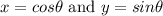
Statement (1) becomes
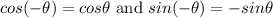
So the value of cos does not change, but the value of sin changes.
Cos is even & sin is odd.
And so sec is even and cosec is odd.
So
 shall be an odd function.
shall be an odd function.
Option B) is the right answer