Question

Ella filled a bucket 7/10 of the way with water. She then poured out 2/10 of the water. How much water remains in the bucket?

Answer 1

Answer: 1/2
----
To do this problem, you'll have to subtract fractions. To subtract fractions, find a common denominator on both fractions (if needed, which you don't need to do for this problem!), subtract the numerators, and put the difference over the common denominator. Simplify if needed.

Ella started out with 7/10 a bucket of water and then lost 2/10 by pouring it out. That means you're finding the difference of 7/10 - 2/10!

1) Find a common denominator
This is already done because both fractions have "10" in the denominator.

2) Subtract the numerators
7 and 2 are the numerators and you're subtracting 2 from 7:
7 - 2 = 5

3) Put the difference of the numerators over the common denominator

`(difference)/(common \:demoninator) = (5)/(10)`

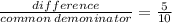
4) Simplify if needed

`(5)/(10) = (1)/(2)`
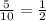
This is because you can divide both the numerator and denominator by 5/5 (and remember 5/5 is the same as 1, and dividing any number by 1 equals itself!)

Answer 2

Answer: The water that remains in the bucket is
`0.56* x`

Step-by-step explanation:

Let the total quantity of water that can be filled in the bucket be x litres

then, volume of water when ella filled it up =
`(7)/(10)* x`

amount of water taken out post that =
`(2)/(10)* (7)/(10)x=(14)/(100x)`

amount of water left in the bucket = amount of water when ella filled it up - amount of water taken out post that =
`(7)/(10)x-(14)/(100)x=(56)/(100)x`

Thus water remains in the bucket is
`0.56* x`