Question

What is the heat needed to raise the temperature of 24.7 kg silver from 14.0 degrees celsius to 30.0 degrees celsius? specific heat capacity of silver is 236 j/(kg * c°). 5.93 e 4 j 8.18 e 4 j 9.32 e 4 j 11.6 e 4 j 17.5 e 4 j?

Answer 1

C

Step-by-step explanation:

9.32 E 4 j

Answer 2

The amount of heat needed to increase the temperature of a substance by
`\Delta T` is given by

`Q=m C_s \Delta T`
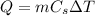
where
m is the mass of the substance

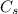
`C_s` is its specific heat capacity

`\Delta T` is the increase of temperature

The sample of silver of our problem has a mass of
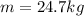
`m=24.7 kg`. Its specific heat capacity is
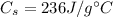
`C_s = 236 J/g^(\circ)C` and the increase in temperature is

`\Delta T=30.0^(\circ)-14.0^(\circ)C=16.0^(\circ)C`

Therefore, the amount of heat needed is

`Q=mC_s \Delta T=(24.7 kg)(236 J/g^(\circ)C)(16.0^(\circ)C)=9.32 \cdot 10^4 J`