Question

What is the sum of the geometric sequence -4, 24, -144 if there are 7 terms

Answer 1

r= -6
-4(1--6^7)/1--6=
-4(279937)/7=
-1119748/7=
-159964

Your answer is A

Answer 2

-159964

Explanation:

Sum of a geometric sequence is given by

S(n) =
`(a(r^(n)-1) )/((r-1))`

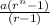
where a = first term of the sequence

r = common ratio

n = number of terms

Sequence is -4, 24, - 144........... 7 terms

here a = (-4)

r =
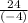`(24)/((-4))` = (-6)

and n = 7

so sum of 7 terms

s (7) =
`((-4)[(-6)^(7)-1] )/((-6-1))`

=
`(4[(-6)^(7)-1] )/(7)`

=
`(4[-279936-1])/(7)`

= -159964