Answer:
The domain of the equation is all real number and the range of the equation is (-∞,-4.885].
Explanation:
The given quadratic equation is
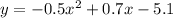
Use a graphing calculator to sketch the graph of the quadratic equation.
The graph of given quadratic equation is shown below.
From the graph it is clear that it is a downward parabola and the vertex of the parabola is (0.7,-4.885).
The function is defined from all values of x, therefore the domain of the function is all real number.
The vertex of the parabola is (0.7,-4.885). It means the maximum value of the function is -4.885 and the value of y cannot be more than -4.885.
So, the range of the function is

Therefore the domain of the equation is all real number and the range of the equation is (-∞,-4.885].