Question

Point A is the incenter of ΔDEF. What is the value of n?

A. 7
B. 11
C. 14
D. 15

help a brotha out.

Thanks!

Answer 1

the answer to your question is B. 11

Answer 2

B. 11

Explanation:

We have been given an image of a triangle and we are asked to find the value of n.

Since we know that in-center of a triangle is the point, where all the angle bisectors of a triangle meet.

Since we have been given that point A is the in-center of ΔDEF. This means the that DN is the angle bisector of angle EDF, so value of
`(3n-6)^(\circ)` will be equal to
`27^(\circ)`

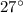
We can represent this information in an equation as:

`(3n-6)^(\circ)=27^(\circ)`

`3n-6=27`

`3n-6+6=27+6`

`3n=33`

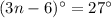
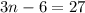
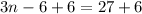
Let us divide both sides of our equation by 3.

`(3n)/(3)=(33)/(3)`

`n=11`

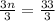
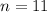
Therefore, the value of n is 11 and option B is the correct choice.