Question

The graph below shows the line of best fit for data collected on the number of cell phones and cell phone cases sold at a local electronics store on twelve different days.

Which of the following is the equation for the line of best fit?

a: y = 0.25x
b: y = 0.5x
c: y = 0.8x
D: y = 0.2x

Answer 1

Option C) y = 0.8x

Explanation:

We are given the following information in the question:

The graph below shows the line of best fit for data collected on the number of cell phones and cell phone cases sold at a local electronics store on twelve different days.

We have to find the equation of line of best fit.

The equation can be calculated with the help of two-point form of equation of straight line.

The equation of line is given by:

`(y-y_1) = \displaystyle(y_2-y_2)/(x_2-x_1)(x-x_1)`

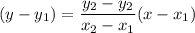
where,
`(x_1,y_1), (x_2.y_2)` is the point through which the line passes.

Taking any two points from the graph, (0,0) and (25,20), we have:

The equation of line is:

`(y-0) = \displaystyle(0-20)/(0-25)(x-0)\\\\y = 2x\\y = (-20)/(-25)x\\\\y = 0.8x`

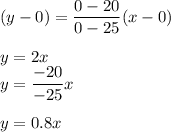
The above equation is the required equation of line of best fit.

Answer 2

C

Explanation:

The equation of a line is given by
`y-y_1=m(x-x_1)`

and

slope (m) is given by:

`m=(y_2-y_1)/(x_2-x_1)`

Where (x_1,y_1) are the first set of point in the line and (x_2,y_2) is the second set of point

Let's take 2 points arbitrarily. (0,0) & (25,20)

Let's plug it and find the equation:

`m=(20-0)/(25-0)=0.8`

Now

`y-y_1=m(x-x_1)\\y-0=0.8(x-0)\\y=0.8x`

C is the correct answer.