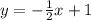Question

A line passes through the points (–6, 4) and (–2, 2). Which is the equation of the line?

Answer 1

`m = (2 - 4)/( - 2 + 6) \\ ( - 2)/(4) = ( - 1)/(2) \\ y - 2 = ( - 1)/(2) (x + 2) \\ y - 2 = - (1)/(2) x - 1 \\ y = - (1)/(2) x + 1`

Answer 2

`y=-(1)/(2)x+1`

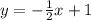
Explanation:

The equation of a line is
`y=mx+b` where m is the pending and b is the y intercept,

First we are going to calculate m:

If you have two points
`A=(x_(1),y_(1))\\B=(x_(2),y_(2))`,

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

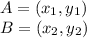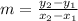
Now we have, A=(-6,4) and B=(-2,2),

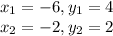`x_(1)=-6 , y_1=4\\x_2=-2, y_2=2` replacing in the formula:

`m=(2-4)/((-2)-(-6)) \\\\m=(-2)/(4) \\\\m=-(1)/(2)`

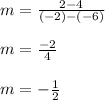
Then
`y=-(1)/(2)x+b`

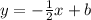
We have to find b, we can find it replacing either of the points in
`y=-(1)/(2)x+b`

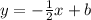
Replacing with (-2,2),

`y=-(1)/(2)x+b\\2=-(1)/(2).(-2)+b\\2=1+b\\2-1=b\\1=b`

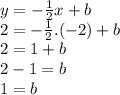
or replacing with (-6,4)

`y=-(1)/(2)x+b\\4=-(1)/(2).(-6)+b\\4=3+b\\4-3=b\\1=b`

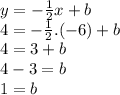
You can see that the result is the same, then the equation of the line is:

`y=-(1)/(2)x+1`