Let
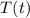
be the temperature of the body

hours after 1:30 PM. Then
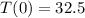
and
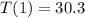
.
Using Newton's Law of Cooling,

, we have
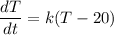
. Now let
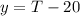
, so
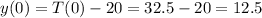
, so

is a solution to the initial value problem
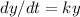
with

.
By separating and integrating, we have

.
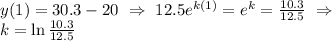
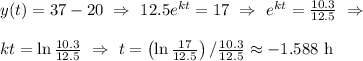
≈ 95 minutes. Thus the murder took place about 95 minutes before 1:30 PM, or 11:55 AM.