Question

I REALLY REALLY REALLY NEED THE ANSWER YOU GUYS THIS IS SO IMPORTANT <3<3<3

WILL GIVE 20 POINTS

Joe went to a fast food restaurant and bought 4 orders of fries and 2 burgers for $14. Becca went to the same fast food restaurant and bought 3 orders of fries and one burger for $9.



Question(s):
1. Write a system of equations that models this situation. Let x represent orders of fries and y represent burgers.
2. Graph the two equations that models this situation and solve the systems using the graph. Let x represent the fries and y represent the burgers and find out how much each order of fries and burgers cost.
3. Use elimination to find out how much an order of fries and a burger cost.
GUYS I REALLY NEED THIS ASAP <3 THANKS SO MUCH
(Please give correct answers)

Answer 1

Part 1:

Let x represent orders of fries and y represent burgers, then the system of equations that models the given situation is given by:


`4x+2y=14 \\ 3x+y=9`


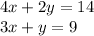
Part 2:

The graph of the two equations is attached.

From the graph it can be seen that the point of intersection of the lines representing the two equations is at point (2, 3), i.e. x = 2, y = 3.

Therefore, each order of fries costs $2 while each order of burger costs $3.


Part 3:

Using elimination, first we make the coefficients of y in both equations by multiplying the second equation by 2 and then subtracting the result from the first equation.

i.e.

equation (2) x 2 gives:


`6x+2y=18`.

Subtracting from equation (1), we have:


`4x-6x=14-18 \\ \\ \Rightarrow-2x=-4 \\ \\ \Rightarrow x= (-4)/(-2) =2`

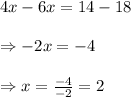
Now, from the second equation,


`y=9-3x \\ \\ =9-3(2) \\ \\ =9-6=3`

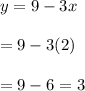
Thus, x = 2 and y = 3.

Therefore, each order of fries costs $2 while each order of burger costs $3.