Answer:
Given the statement: if y =3x+6.
Find the minimum value of
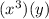
Let f(x) =
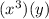
Substitute the value of y ;
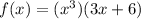
Distribute the terms;
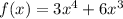
The derivative value of f(x) with respect to x.
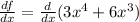
Using
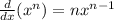
we have;

Set
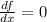
then;
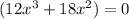

By zero product property;
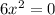 and 2x + 3 = 0
and 2x + 3 = 0
⇒ x=0 and x =

then;
at x = 0
f(0) = 0
and
x = -1.5
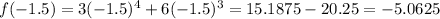
Hence the minimum value of
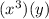 is, -5.0625
is, -5.0625