Question

Compare each of the functions shown below: f(x) graph of a downward facing parabola with vertex at 2, 3 g(x) = 2 cos(2x − π) + 2 h(x) x y −1 −7 0 −2 1 1 2 2 3 1 4 −2 5 −7 Which function has the largest maximum

Answer 1

h(x) has the largest

hope this helps

Answer 2

Hence, the function g(x) has the largest maximum.

Explanation:

• Since f(x) is a downward facing parabola with vertex at (2,3).

Since the parabola is downward facing so the vertex is the point of the maximum.

Hence the maximum value of f(x) is 3 at x=2.

• Now we are given a function g(x) as:

`g(x)=2 \cos (2x-\pi)+2`

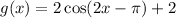
Since cosine function can take a maximum value of 1 so the maximum value of the function g(x) that can exist is: 2×1+2=4.

Hence, maximum value of g(x) is:3

• Now the function y=h(x) is defined as:

x y

-1 -7

0 -2

1 1

2 2

3 1

4 -2

5 -7

The largest value of function h(x) is 2.

Hence, the function g(x) has the largest maximum.