Question

A central angle measuring 120° intercepts an arc in a circle whose radius is 6. What is the area of the sector of the circle formed by this central angle?

Answer choices:
1/3π
12π
24π
36π

Answer 1

thirddd oneeee..........

Answer 2

Answer:
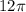`12\pi` square units

Explanation:

Area of sector is given by :

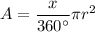`A=(x)/(360^(\circ))\pi r^2` , where x is the central angle made by arc and r is the radius of the circle .

As per given , we have

`x=120^(\circ)`

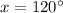
Radius = 6 units

Then, the area of the sector made by arc will be :

`A=(120^(\circ))/(360^(\circ))\pi (6)^2\\\\=(1)/(3)\pi (36)=12\pi`

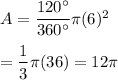
Hence, the area of the sector of the circle formed by this central angle =
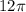`12\pi` square units.