Answer:
Domain of f(x) is { x : x ∈ R and x > -6 }
Explanation:
Given function is,
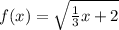
We need to find Domain of the function.
The domain of a function is the complete set of possible values of the independent variable.
Domain of f(x) is set of real number excluding point where value under square root becomes negative.
So, to find that point
put ,
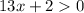
x + 6 > 0
x > -6
So, Value of x must be greater than -6
⇒ Domain of f(x) = { x : x ∈ R and x > -6 }
Therefore, Domain of f(x) is { x : x ∈ R and x > -6 }