Answer:
Second quadrant.
Explanation:
The given angle is
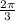
To know where this angle terminates, first, we need to transform it into degrees.
We know that
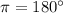
So,
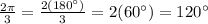
Now, each quadrant is delimited by an angle interval:
- I quadrant from 0° to 90°.
- II quadrant from 90° to 180°.
- III quadrant from 180° to 270°.
- IV quadrant from 270° to 360°.
Our angle is 120°, that means it terminates inside the second quadrant, because it's determined by the range 90° - 180°, and 120° is inside.
Therefore, the given angle terminates in the second quadrant.