Question

The area of a room is 396 square feet. The length is (x + 8) feet and the width is (x + 4) feet. Find the dimensions of the room.

You only need to find the length and width.

Answer 1

L:18ft
W:22ft
Hope it helps

Answer 2

Area of rectangle (A) is given by:

`A = lw`

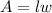
where, l is the length and w is the width of the rectangle.

As per the statement:

The area of a room is 396 square feet.

The length is (x + 8) feet and the width is (x + 4) feet.

⇒A = 396 square feet , l = (x+8) feet and w = (x+4) feet

Substitute these given values we have;

`396 = (x+8)(x+4)`

⇒
`396 = x^2+4x+8x+32`

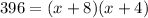
Combine like terms;

⇒
`396 = x^2+12x+32`

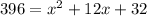
Subtract 32 from both sides we have;

`364 = x^2+12x`

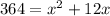
or

`x^2+12x-364 =0`

⇒
`x^2+26x-14x-364 = 0`

⇒
`x(x+26)-14(x+26)=0`

⇒
`(x+26)(x-14) = 0`

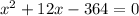
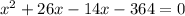
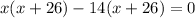
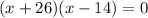
By zero product property we have;

x+26 = 0 or x-14 = 0

⇒x = -26 and x = 14

Since, side cannot be in negative.

⇒x = 14

then;

l = x+8 = 14+8 = 22 feet

w = x+4 = 14+4 = 18 feet

Therefore, the dimensions of the room are:

l = 22 feet and w = 18 feet