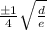Answer:
The value of f out of given expression
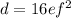 is
is
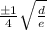
Explanation:
Given : expression
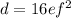
We have to solve for f.
Consider the given expression
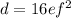
Divide both side by 16e, we get,
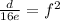
Now, taking square root, both sides, we have,
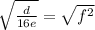
Simplify, we get,
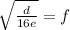
We know
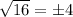 , we get,
, we get,
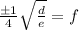
Thus, The value of f out of given expression
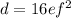 is
is