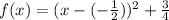Answer:
The function in vertex form is
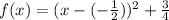
Explanation:
Given: Function
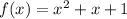
We have to write the given function
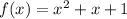 in vertex form.
in vertex form.
Consider the given function
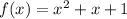
Using algebraic identity
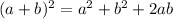
Comparing a = x , 2ab = x
Also, 2b = 1 ⇒

Add and subtract
 , we get,
, we get,
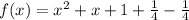
Simplify, we have,
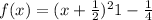
Simplify, we have,
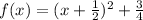
Thus, The function in vertex form is