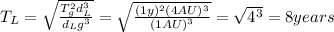Answer:
8 years
Step-by-step explanation:
Kepler's third law states that the ratio between the cube of the distance of a planet from its star and the square of its orbital period is constant for all the planets orbiting around that star:
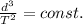
where d is the distance of the planet from the star and T is the orbital period.
By applying this law to the two planets of this problem, we can write

where
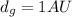 is the distance of geos from the star,
is the distance of geos from the star,
 is its orbital period,
is its orbital period,
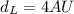 is the distance of logos from the star. Re-arranging the equation , we can find
is the distance of logos from the star. Re-arranging the equation , we can find
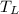 , the orbital period of logos around the star:
, the orbital period of logos around the star: