Question

Select the graph for the solution of the open sentence. Click until the correct graph appears. 5|x| + 3 < 18

Answer 1

The correct answer is the second graph. It is the one with open circles at -3 and 3 and a line connecting them.

To find this, you just solve the equation like a regular equation. Subtract 3 from both sides and then divide by 5.

You will get that the absolute value of x is less than -3. Therefore, x must be in between -3 and 3.

Answer 2

The correct option is 2.

Explanation:

The given inequality is

`5|x|+3<18`

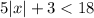
Subtract 3 from both the sides.

`5|x|+3-3<18-3`

`5|x|<15`

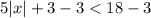
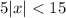
Divide both sides by 5.

`|x|<(15)/(5)`

`|x|<3`

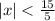
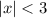
If |x|<a, then the solution set is -a<x<a.

`-3<x<3`

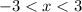
-3 and 3 are not included in the solution set because the sign of inequality is <. So, there are open circle at x=3 and x=-3.

Only graph 2 represents the solution set.

Therefore the correct option is 2.