Question

(09.05 MC) Derek kicks a soccer ball off the ground and in the air, with an initial velocity of 31 feet per second. Using the formula H(t) = −16t2 + vt + s, what is the maximum height the soccer ball reaches? (5 points) a:14.2 feet b:14.6 feet c:15.0 feet d:15.3 feet

Answer 1

In order to find the maximum of the function, we have to find the roots of the first derivative.

`H(t)=-16t^2+vt+s\\ H'(t)=-32t+v=0\\ v=32t\\ t=(v)/(32)=(31)/(32)=0.97s`
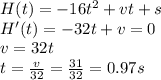
This gives us the time at which the ball reaches its maximum height.
We don't know s. We can find it because we know that at t=0 the ball is on the ground and its height has to be zero.

`H(0)=0=-16(0)^2+v(0)+s\\ s=0`
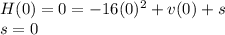
Finally, we can find the maximum height:

`H(0.97)=-16(0.97)^2+31\cdot 0.97=15.0156 \simeq15$ft`
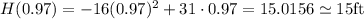
The answer is C.