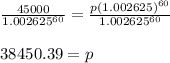Question

Marilyn knows that she needs $45,000 for a down payment on a house. She found an investment that earns 3.15% interest compounding monthly. She would like to purchase the home in 5 years. How much should she put in the account now to ensure she has her down payment?

$38,450.39

$30,871.96

$44,296.89

$52,665.27


Can you explain how to do it? Thanks :)

Answer 1

She should save $38,450.39.

The formula for the amount of money in an interest-bearing account that is compounded is


`A=p(1+(r)/(n))^(nt)`

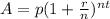
where A is the total amount in the account, p is the amount of principal invested, r is the interest rate as a decimal number, n is the number of times per year interest is compounded, and t is the number of years. Using our information we have:


`45000=p(1+(0.0315)/(12))^(12*5) \\ \\45000=p(1.002625)^(60)`

Divide both sides:

`(45000)/(1.002625^(60))=(p(1.002625)^(60))/(1.002625^(60)) \\ \\38450.39=p`