Question

Need help ASAP please thankyou!

Answer 1

c=7

d=5

Explanation:

Exponential Equations

We need to recall some properties of exponentials to solve the equations:

`x^a*x^b=x`{a+b}`

`\displaystyle (x^a)^b=x^(ab)`

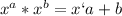
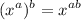
Equation A

`√(448x^c)=8x^3√(7x)`

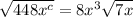
Squaring:

`(√(448x^c))^2=(8x^3√(7x))^2`

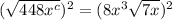
Simplifying the radicals:

`448x^c=64x^6(7x)`

`448x^c=448x^7`

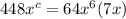
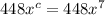
If follows that

c=7

Equation B

`\sqrt[3]{576x^d}=4x\sqrt[3]{9x^2}`

Raising to the third power:

`(\sqrt[3]{576x^d})^3=(4x\sqrt[3]{9x^2})^3`

`576x^d=4^3x^3(9x^2)`

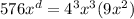
Operating

`576x^d=64x^3(9x^2)=576x^5`

Thus:

d=5