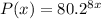Answer:
A)
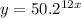
B)
Explanation:
Since, the population of bacteria after x days who are growing with a constant factor is,
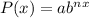
Where a is the initial population of bacteria
b is the growth factor per period,
n is the number of periods in a day,
Given,
A) In Colby’s experiment,
The initial number of bacteria, a = 50,
Also, they are doubling in every 2 hours.
⇒ Number of period, n = 24/2 = 12, ( 1 day = 24 hours )
Growth factor, b = 2
Thus, the population of bacteria after x days is,
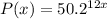
B) In Jaquan’s experiment ,
The initial number of bacteria, a = 80,
Also, they are doubling in every 3 hours,
⇒ Number of period, n = 24/3 = 8,
Growth factor, b = 2
Thus, the population of bacteria after x days is,