Question

Write an equivalent expression for 1/y-1/4

Answer 1

We have the expression
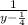
`(1)/(y- (1)/(4) )`. To write a equivalent expression, we are going to rewrite the denominator of our expression using its common denominator 4:

`(1)/(y- (1)/(4) ) = (1)/( (4y-1)/(4) )`
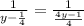
Now, notice that we are basically diving 1 by
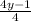
`(4y-1)/(4)`, so we have a division involving fractions, and remember that to divide fractions, we just need to invert the second fraction and multiply:

`(1)/( (4y-1)/(4) ) =1* (4)/(4y-1) = (4)/(4y-1)`

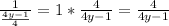
We can conclude that the equivalent expression of
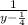
`(1)/(y- (1)/(4) )` is
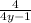
`(4)/(4y-1)`.