Question

Can you exchange the coordinates of points (x1,y 1) and (x2, y2) in the distance formula and still find the correct distance? Explain your answer with an example.

Answer 1

It doesn't matter because, in the end, the differences are squared and multiplying a negative by a negative yields a positive.

Answer 2

Yes. we can exchange the coordinates.

Explanation:

Distance formula says that

distance between

`(x_1, y_1) & (x_2, y_2) = √((x_2-x_1)^2+(y_2-y_1)^2)`

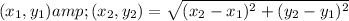
and we consider the positive root only

If these two are interchanged when we square the result would be the same.

Example:

(1,2) and (-2, -2)

Distance in one order =
`√((1+2)^2+(2+2)^2) =5`

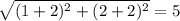
If order is interchanged

distance =
`√((-2-1)^2+(-2-2)^2) =5`

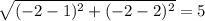
Thus we find that order does not matter while calculating distance.