Answer:
Option A is the correct answer.
Explanation:
y intercept means x value is 0.
f(0) = 3 x 0 + 4 = 4
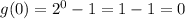
We need same y intercepts
That is f(0) = g(0)
4 + a = 0 + b
Checking all the options
a) f(x) - 3 and g(x) + 1
4 - 3 and 0 + 1
1 and 1
Option A is correct.
b) f(x) + 4 and g(x) - 1
4 + 4 and 0 - 1
8 and -1
Option B is wrong.
c) f(x) - 2 and g(x) + 1
4 - 2 and 0 + 1
2 and 1
Option C is wrong.
d) f(x) + 3 and g(x) - 6
4 + 3 and 0 - 6
7 and -6
Option D is wrong.
So, Option A is the correct answer.