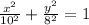Question

I need help ASAP pleaaaaaase

What is the equation of an ellipse whose foci at (+/-6,0) and c/a=3/5

Answer 1

Consider this option:
the equation of ellipse is: x²/a² + y²/b² = 1
1. if focuses are (6;0) and (-6;0), it means, that 2c=6-(-6)=12, ⇒ c=6.
2. c²=a²-b², it means, that a²-b²=36 - this is the 1st equation.
3. if c/a=3/5, it means, that c²/a²=9/25, ⇒ (a²-b²)/a²=9/25 - this is the 2d equation.
4. the 1st and the 2d equations are the system of two equations:

`\left \{ {{a^2-b^2=36} \atop { (a^2-b^2)/(a^2)= (9)/(25)}} \right. \ =\ \textgreater \ \ \left \{ {{a^2=100} \atop {b^2=64}} \right.`
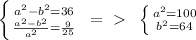
5. the required equation of the ellipse is:

`(x^2)/(10^2) + (y^2)/(8^2) =1`