Question (6)
To answer this question we will find out both probabilities one by one and then we will multiply both probabilities.
Probability of getting a number card from standard deck will be
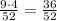
Probability of getting one ace will be
 as we are given that second card is drawn without replacement.
as we are given that second card is drawn without replacement.
To find the probability of choosing a number card and then an ace without replacement will be,
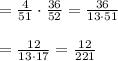
Therefore, first option is the correct choice.
Question (7)
Let us find out probability that Shironda will get an even number after rolling a cube. We know that a standard dice contains 3 even and 3 odd numbers.
Probability of getting an even number =
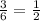 .
.
Now let us find out probability of getting a black card from the deck.
Probability of getting a black card,
=
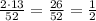
Now we can get probability of getting an even number and a black card by multiplying both probabilities.
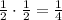
Therefore, probability of getting an even number and a black card is 1/4.