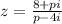
given Re(z)=2/5
Part (a) to find the possible values of p.
1. we rationalize z by multiplying the denominator by it's conjugate
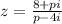

]multiple top & bottom by conjugate of denominator]


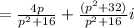
2. The real part is therefore
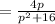
and we have been given that Re(z)=2/5.
We now form the equation
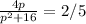
which transforms to the quadratic equation
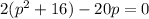
and simplifies to
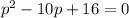
and factors to
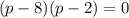
and using the zero product property, we deduce that
p=8 or p=2
Check:
substitute p=8 in z gives
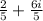
....good
substitute p=2 in z gives
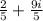
....good
If you need help with the other parts, please let me know.